Quantum computing promises to revolutionize fields ranging from cryptography to materials science, but it faces several fundamental challenges. While issues like qubit stability, error correction, and scalability often dominate discussions, an equally critical yet less widely recognized challenge is the fidelity and speed of quantum measurements.
In classical computing, measuring a system’s state is straightforward and nearly instantaneous. However, in quantum computing, measurement is a complex process that disrupts the quantum state. This makes fast and accurate quantum measurements crucial for the efficiency of quantum algorithms and error correction protocols. A recent study published in Physical Review Letters proposes a novel solution to this challenge—trading space (additional qubits) for time—to significantly speed up quantum measurements while maintaining or improving their accuracy.
A Breakthrough in Quantum Measurement Speed
Led by Christopher Corlett, Professor Noah Linden, and Dr. Paul Skrzypczyk from the University of Bristol, in collaboration with researchers from the University of Oxford, the University of Strathclyde, and Sorbonne Université, the study introduces an innovative approach to reducing measurement times in quantum computing. Their technique involves using ancillary qubits—additional qubits entangled with the target qubit—to amplify the amount of information extracted in a fixed period.
Quantum measurements typically require prolonged interaction with a qubit to achieve higher accuracy. However, longer measurement times introduce noise and decoherence, leading to errors and making quantum error correction more difficult. The proposed space-time trade-off method addresses this issue by leveraging extra qubits to distribute measurement load, achieving the same accuracy in a fraction of the time.
Dr. Skrzypczyk emphasizes the importance of this advancement, stating, “Accurate and fast quantum measurements are crucial for emerging quantum technologies. Our approach allows for faster and more reliable error correction, which is essential for building scalable and fault-tolerant quantum computers.”
Understanding the Measurement Challenge
At the heart of quantum computing is the challenge of measuring qubits without introducing significant error. Qubits exist in superposition, meaning they can be in a state of 0, 1, or both simultaneously. However, when measured, they collapse into one of these states, losing quantum coherence.
To understand the need for prolonged measurements, consider a simple analogy: Suppose you are shown two nearly identical glasses of water—one containing 100 ml and the other 90 ml. If you only get a brief glance, distinguishing the difference is difficult. However, if you observe them longer, you gain confidence in your assessment.
The same principle applies to quantum measurements. The longer a qubit is observed, the more information can be extracted, leading to a more accurate reading. However, this approach introduces delays and noise, making it unsuitable for real-time quantum operations. The research team’s approach circumvents this limitation by amplifying the information extracted without extending the measurement time.
The Role of Ancillary Qubits in Measurement Enhancement
The key to speeding up quantum measurements lies in ancillary qubits—additional qubits entangled with the target qubit. These ancillary qubits serve as information amplifiers, allowing measurements to be conducted more quickly without sacrificing accuracy.
Professor Corlett explains this concept using the water glass analogy: “If instead of looking at a 10 ml difference, you scale up both glasses to 200 ml and 180 ml, the difference becomes easier to detect in less time. By introducing an additional ancillary qubit, we effectively double the volume difference. With more ancillary qubits, the observation time required continues to shrink.”
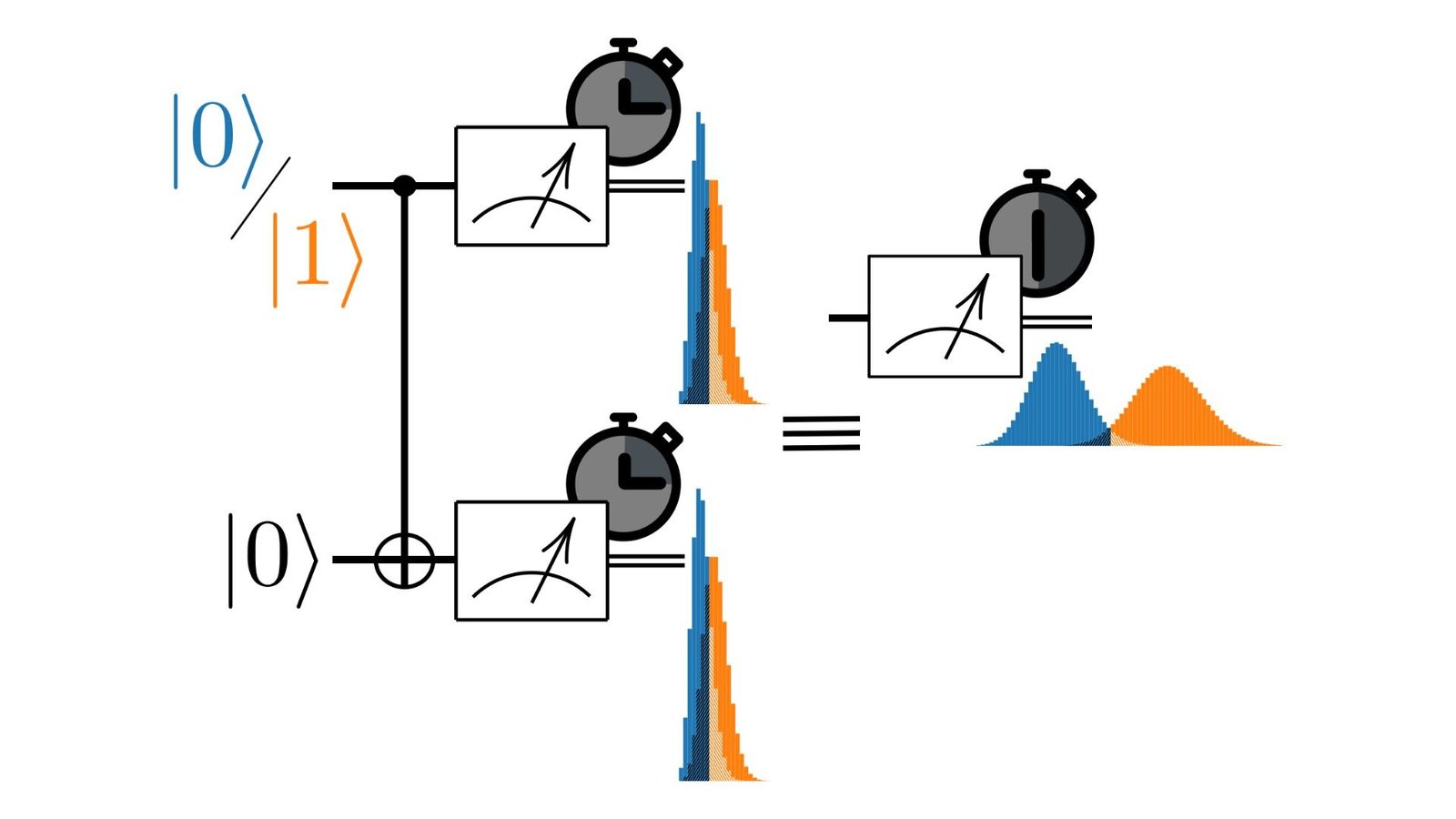
In practical terms, the research team demonstrated that if a single qubit requires five seconds to measure accurately, using five qubits simultaneously would achieve the same level of accuracy in just one second. This represents a linear speedup, a significant breakthrough for real-time quantum computing applications.
The Space-Time Trade-Off Mechanism
The core of the researchers’ technique builds on previous quantum error correction methods, particularly repetition codes. In their approach, the target qubit is entangled with N-1 ancillary qubits, and the information from the target qubit is copied onto these ancillary qubits using controlled quantum operations known as CNOT (Controlled-NOT) gates.
Instead of measuring the target qubit for a long duration, all N qubits (including ancillary ones) are measured simultaneously for a proportionally shorter time (t/N instead of t). By summing the measurement results, the system achieves the same level of confidence as a longer single-qubit measurement, effectively trading spatial resources (qubits) for temporal efficiency.
According to Professor Corlett, “This technique allows us to speed up quantum measurements without compromising their quality. By distributing the measurement process across multiple entangled qubits, we can reduce noise and improve error correction efficiency.”
Real-World Applications and Experimental Validation
Beyond theoretical validation, the researchers tested their method against various noise models to evaluate its robustness in practical quantum computing environments.
In ideal noise-free conditions, the space-time trade-off scheme demonstrated a perfect linear speedup, meaning that doubling the number of qubits halved the required measurement time. More impressively, in realistic noisy conditions, the method not only maintained its efficiency but, in some cases, outperformed expectations by providing better-than-linear speedup.
Professor Linden highlights the practical importance of noise resilience, stating, “Ensuring our scheme works under realistic noise conditions is crucial for its practical implementation. Any breakthrough in quantum computing must be robust against noise to be truly useful in real-world systems.”
The scheme is designed to be adaptable to multiple quantum computing platforms, including superconducting qubits, trapped ions, and cold atom-based quantum processors. These are the leading architectures in the race to build scalable quantum computers, and the ability to enhance measurement efficiency across these systems makes the proposed method highly valuable.
Future Directions and Experimental Implementation
While the study presents a strong theoretical foundation, the next step involves experimental implementation. The researchers are now collaborating with quantum hardware developers to test their method on physical quantum systems. If successful, this technique could be integrated into future quantum computing architectures, accelerating progress toward fault-tolerant quantum computers.
Dr. Skrzypczyk notes that the long-term impact of this work could be profound: “Our approach could significantly improve the speed and efficiency of quantum error correction, bringing us closer to practical, large-scale quantum computers. We look forward to seeing how it performs in experimental settings.”
A Step Closer to Practical Quantum Computing
The ability to perform fast and accurate quantum measurements is a crucial step toward making quantum computing viable for real-world applications. The space-time trade-off method proposed by the researchers offers a promising solution to one of quantum computing’s fundamental challenges.
By leveraging additional qubits to distribute measurement tasks, their approach provides a path to faster quantum operations without compromising accuracy. As experimental validation progresses, this breakthrough could reshape the way quantum measurements are conducted, paving the way for more efficient and scalable quantum technologies.
With continued advancements in measurement techniques, quantum computing moves closer to realizing its full potential—offering unprecedented computational power for solving some of the world’s most complex problems.
Reference: Christopher Corlett et al, Speeding Up Quantum Measurement Using Space-Time Trade-Off, Physical Review Letters (2025). DOI: 10.1103/PhysRevLett.134.080801.