10 Most Important Chemistry Formulas (And How to Use Them)

Chemistry is more than a subject taught in classrooms. It’s the quiet architect behind every experience you have—cooking, breathing, traveling, even falling in love. And like any architect, chemistry builds its intricate structures with a set of powerful blueprints: formulas.

Formulas are the compact expressions of massive ideas. They turn the chaos of atoms and molecules into something predictable, controllable, and even beautiful. Imagine trying to bake without measurements or travel without a map—this is what trying to do chemistry without formulas would be like.

In this article, we’ll explore ten of the most essential chemistry formulas, understand not just what they are, but how and why to use them. You won’t just memorize them; you’ll see them come alive through real-world applications, colorful stories, and practical examples that showcase why chemistry’s formulas are some of humanity’s most magical inventions.

Let’s dive deep.

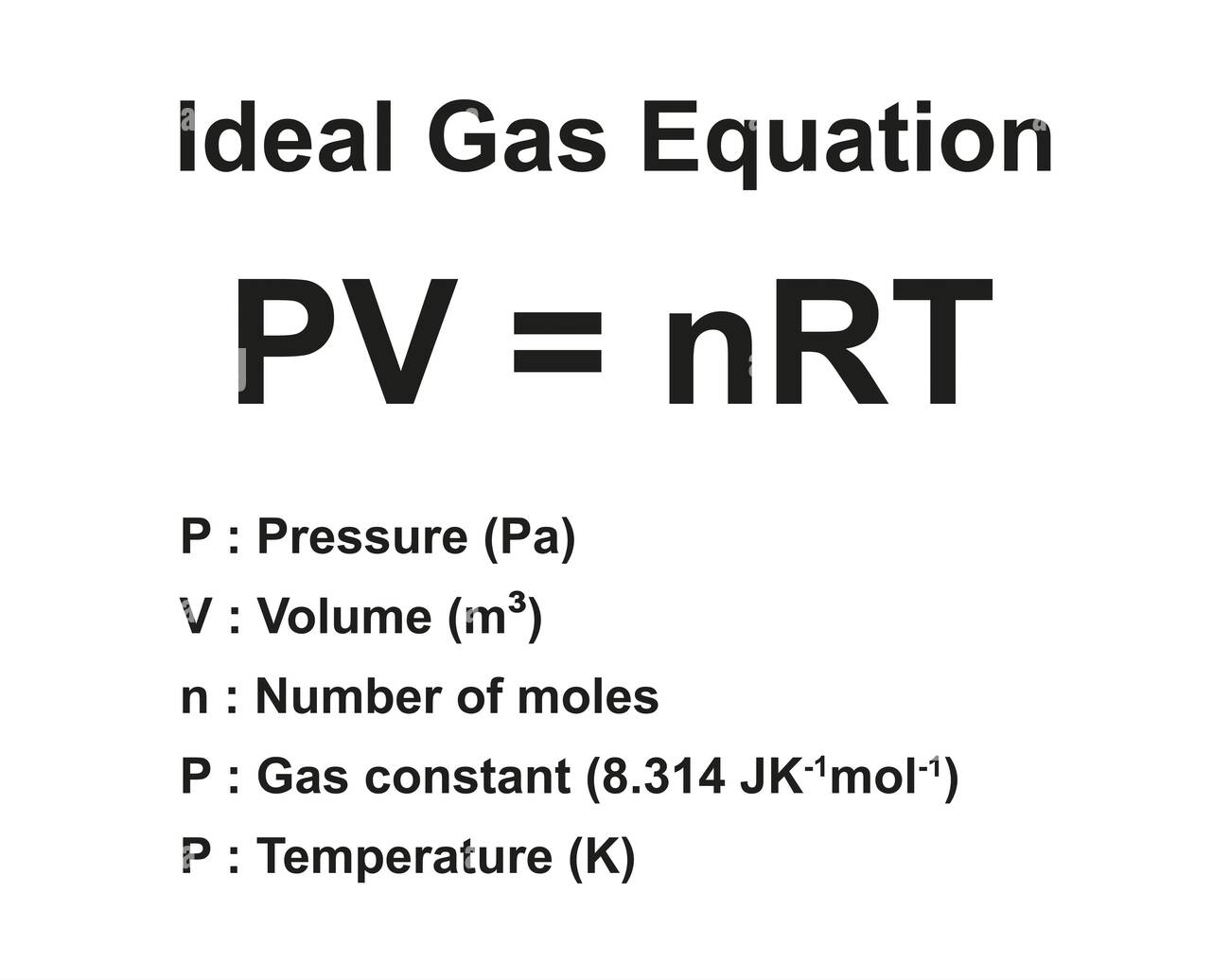
1. The Ideal Gas Law — PV = nRT

The Ideal Gas Law is chemistry’s Swiss army knife for gases. It elegantly ties together pressure (P), volume (V), number of moles (n), temperature (T), and the universal gas constant (R) into a single, versatile formula.

In practical terms, it explains everything from why your car tires inflate in hot weather to how astronauts breathe on the International Space Station.

Suppose you want to calculate the volume occupied by 3.00 moles of an ideal gas at a temperature of 300 Kelvin and a pressure of 2.00 atm. Using R = 0.0821 L·atm/mol·K:

V = (nRT) / P

Plugging in:

V = (3.00 mol)(0.0821 L·atm/mol·K)(300 K) / (2.00 atm) = 36.945 L

Just like that, you’ve calculated how much space your gas will occupy.

This formula is fundamental in fields from engineering to meteorology and space science. The beauty of PV = nRT is how it makes the unpredictable nature of gas particles wonderfully predictable.

2. The Law of Conservation of Mass — Mass of Reactants = Mass of Products

Centuries ago, people believed that substances could simply vanish into thin air. Then came Antoine Lavoisier, who performed careful experiments and boldly declared that mass is conserved.

When you burn a log, the ash you see is far less massive than the original wood—but if you trap the gases released, the total mass is identical.

This law is the foundation of chemical equations. In practice, if you have a chemical reaction:

2 H₂ + O₂ → 2 H₂O

and you start with 8.0 grams of hydrogen and 64.0 grams of oxygen, you’ll form exactly 72.0 grams of water vapor.

This principle ensures industrial reactions produce consistent yields. It underpins pharmaceutical manufacturing, environmental monitoring, and even forensic science. Without it, chemistry would be chaos.

3. The Molarity Formula — M = moles of solute / liters of solution

Imagine making lemonade. The sweetness depends on the ratio of sugar to water—too little sugar, and it’s sour; too much, and it’s syrupy. Chemistry formalizes this concept with molarity.

Molarity measures how concentrated a solution is. To prepare a 1.0 M NaCl solution, dissolve 58.44 grams (one mole) of salt into one liter of water.

Molarity makes laboratory life possible. Chemists calculate exactly how much reactant they need to start a reaction. Surgeons depend on saline solutions of precise molarity during operations. Even astronauts’ hydration packs are carefully crafted to maintain electrolyte balance, thanks to this formula.

Mastering molarity means mastering control over chemical environments.

4. Percent Composition Formula — % Composition = (Mass of Element / Molar Mass of Compound) × 100

How much oxygen is there in water? How much carbon is packed into sugar?

Percent composition allows chemists to break down a compound and understand what proportion of it is made up by each element.

Take water (H₂O). The molar mass is approximately 18.02 g/mol. Two hydrogens contribute about 2.02 grams, and oxygen about 16.00 grams.

Thus, the percent composition of oxygen:

%O = (16.00 / 18.02) × 100 ≈ 88.79%

And hydrogen:

%H = (2.02 / 18.02) × 100 ≈ 11.21%

Understanding percent composition is crucial for industries like pharmaceuticals, where a tiny error in ingredient proportions can mean the difference between medicine and poison.

5. Empirical Formula Calculation — Find the simplest whole number ratio

Suppose you discover a mysterious compound that contains 40% carbon, 6.7% hydrogen, and 53.3% oxygen by mass. How do you find its formula?

Start by assuming 100 grams of the substance. That gives you:

• 40 grams C → 3.33 moles
• 6.7 grams H → 6.7 moles
• 53.3 grams O → 3.33 moles

Divide each by the smallest number of moles:

• C: 3.33 / 3.33 = 1
• H: 6.7 / 3.33 ≈ 2
• O: 3.33 / 3.33 = 1

Thus, the empirical formula is CH₂O—formaldehyde.

Empirical formulas are the detective work of chemistry, teasing out the secrets of unknown substances.

6. Avogadro’s Number — 6.022 × 10²³ Particles per Mole

Avogadro’s number is awe-inspiring. One mole of any substance contains 6.022 × 10²³ particles—atoms, molecules, or ions.

To grasp this, imagine if you had a mole of sand grains. It would cover the Earth many times over to a depth of several meters. A mole is the chemist’s way of dealing with atoms and molecules, quantities so tiny that counting them individually would be absurd.

If you have 2 moles of oxygen molecules, you possess:

2 × 6.022 × 10²³ = 1.2044 × 10²⁴ molecules

Avogadro’s number is a bridge between the atomic and macroscopic worlds. It turns the unimaginable into the manageable.

7. The Dilution Formula — M₁V₁ = M₂V₂

Suppose you have a 6.0 M solution of hydrochloric acid, but you need 250 mL of 1.0 M solution for an experiment.

Using the dilution formula:

M₁V₁ = M₂V₂

(6.0 M)(V₁) = (1.0 M)(0.250 L)

V₁ = 0.0417 L = 41.7 mL

Thus, you take 41.7 mL of the concentrated acid and dilute it with water to make 250 mL.

This formula is vital in preparing safe concentrations for laboratory work, medical applications, and food manufacturing. It allows for flexibility and precision in chemical handling.

8. Gibbs Free Energy — ΔG = ΔH – TΔS

One of the grand questions in chemistry is whether a reaction will happen spontaneously. Gibbs Free Energy provides the answer.

ΔG represents the maximum reversible work a system can perform. It balances the change in enthalpy (ΔH, heat energy) and entropy (ΔS, disorder), factoring in temperature (T).

If ΔG < 0, the reaction is spontaneous. If ΔG > 0, it’s non-spontaneous.

For example, the rusting of iron is spontaneous because it releases energy and increases disorder. Freezing water at room temperature is non-spontaneous because it would require removing heat unnaturally.

Gibbs Free Energy powers understanding of chemical kinetics, thermodynamics, and biological processes, from enzyme action to human metabolism.

9. Beer-Lambert Law — A = εlc

Chemistry meets light in the Beer-Lambert Law, which relates the absorbance (A) of light by a solution to the concentration (c) of the absorbing species, the path length (l) of light through the solution, and the molar absorptivity (ε).

Spectrophotometers use this principle daily. Measuring the absorbance at a specific wavelength reveals the concentration of DNA in a solution or pollutants in a river.

Suppose a sample has ε = 50 L/(mol·cm), l = 1 cm, and A = 0.5. The concentration c is:

c = A / (εl) = 0.5 / (50 × 1) = 0.01 mol/L

The Beer-Lambert Law unlocks everything from forensic toxin analysis to quality control in food production.

10. Henderson-Hasselbalch Equation — pH = pKa + log([A⁻]/[HA])

Buffer solutions are the unsung heroes of biology and chemistry. Your blood’s pH is maintained within a narrow range thanks to buffering.

The Henderson-Hasselbalch Equation helps predict the pH of buffer solutions. If you know the concentration of an acid (HA) and its conjugate base (A⁻), you can calculate the pH:

pH = pKa + log([A⁻]/[HA])

For example, if acetic acid (pKa = 4.76) is in a solution where [A⁻] = 0.1 M and [HA] = 0.1 M:

pH = 4.76 + log(0.1/0.1) = 4.76 + log(1) = 4.76

Maintaining pH stability is critical in pharmaceuticals, environmental monitoring, and industrial processes.

Conclusion: Chemistry’s Formulas—Keys to Understanding the Universe

These ten formulas are more than abstract concepts—they are the foundation stones of chemistry. Each one condenses volumes of understanding into a simple, usable form. They let scientists predict reactions, create new materials, heal the sick, feed billions, and even explore the stars.

Learning these formulas is not about memorization; it’s about seeing the hidden order of the universe. With them, you step into the shoes of chemists past and present, joining the grand tradition of those who seek to understand, transform, and elevate the world around us.

So next time you stir sugar into your coffee, gaze at a fireworks show, or wonder how your smartphone battery works, remember: behind the magic lies a formula, and behind the formula, an invitation to explore deeper still.